2 Sigma Calculation for Data Analysis in Excel – Step by Step Guide

When it comes to data analysis, the concept of sigma (σ), also known as standard deviation, plays a crucial role in understanding variability. Sigma measures the spread or dispersion of a dataset, and calculating 2 sigma can help me identify data points that are two standard deviations away from the mean. In this guide, I’ll walk through how to calculate 2 sigma in Excel, explaining the process in a clear, step-by-step manner.

5 Key Takeaways

• 2 Sigma Defined: It represents two standard deviations from the mean, covering 95% of data points in a normal distribution.
• Practical Use: Helps identify outliers and gauge data consistency.
• Calculation in Excel: Use the formulas =AVERAGE() and =STDEV.S() to calculate the mean and standard deviation.
• Applications in Manufacturing: 2 sigma is used to ensure consistent quality in products.
• Risk Management: In finance, it helps measure volatility and assess investment risks.

 

Unlocking the Mysteries of Sigma in Excel

What Is 2 Sigma and Why It Matters?

Navigating the landscape of statistical analysis in Excel, we encounter the concept of 2 sigma. In statistical terms, 2 sigma represents a measure of variability or spread of a dataset. This is useful when I want to filter out outliers or assess the variability of a dataset.

In the context of a normal distribution, 1 sigma (one standard deviation) from the mean covers about 68% of the data. When I extend to 2 sigma, it means I’m looking at roughly 95% of the data that falls within two standard deviations from the mean.

Understanding 2 sigma is crucial because it provides deep insights into the consistency and reliability of processes or datasets we’re analyzing. It indicates how much we can expect results to deviate from the average and serves as a foundational element in crafting control limits for quality assurance.

The Role of Standard Deviation in Data Analysis

Standard deviation is a powerhouse metric in data analysis, primarily because it lets us quantify the degree of variation in our data. It shines a light on how densely packed or widely spread the data points are around the mean.

When we dig into datasets, especially those that are expected to follow a normal distribution, standard deviation becomes our compass for navigating data scatter. It answers questions like, “Are these values consistent?” or “How predictable is this process?”

For us as data analysts, standard deviation also acts as a risk assessment tool. In finance, for instance, it can signal high volatility in stock prices, while in manufacturing, a small standard deviation implies high-quality control with consistent product output.

Through understanding and applying this measure, we bolster our data storytelling, providing a narrative that captures the essence of variability and what that means for decision-making and strategic planning.

 

Step-by-Step Guide to Calculate 2 Sigma

STEP 1: Organizing the Data in Excel

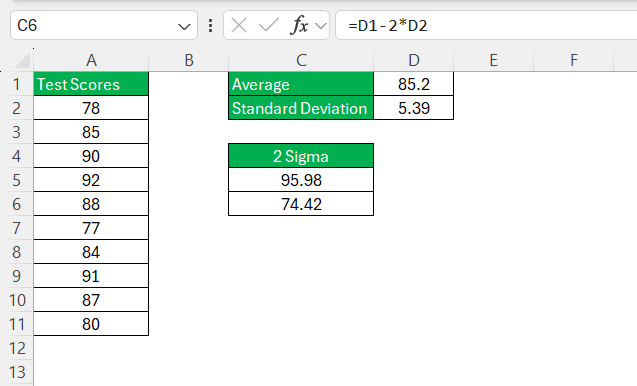
Open Excel and input the data I want to analyze. For example, I’ll use test scores like: 78, 85, 90, 92, 88, 77, 84, 91, 87, 80. Enter this data into a column, starting in cell A2.

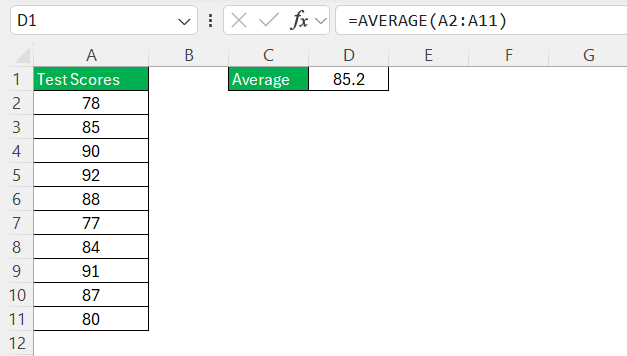
STEP 2: Calculate the Mean

In an empty cell, type the formula =AVERAGE(A2:A11) to calculate the average of the values in cells A2 through A11. Press Enter. For this example, the mean might be 85.2.

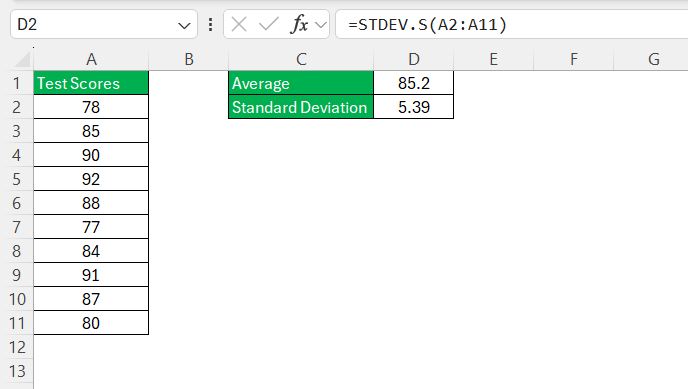
STEP 3: Calculate the Standard Deviation (Sigma)

In another empty cell, use the standard deviation function =STDEV.S(A2:A11) to calculate the standard deviation. This is useful for a sample of data. For my dataset, the standard deviation might be 5.39.

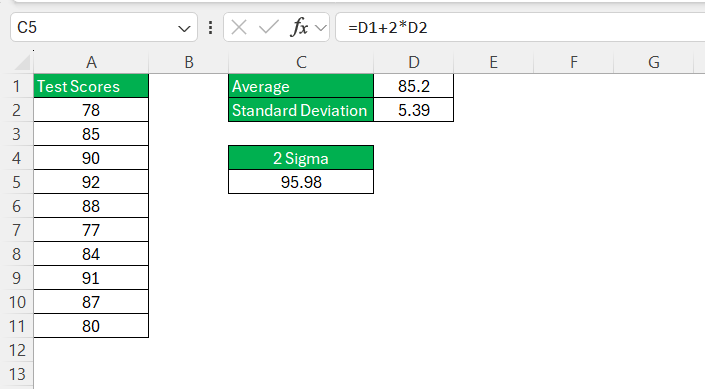
STEP 4: Calculate 2 Sigma

In a new cell, type =D1 + 2 * D2 to calculate the upper bound (Mean + 2 Sigma). For my data, this might be 95.98.

Next, type =D1 - 2 * D2 in another cell to calculate the lower bound (Mean – 2 Sigma). This might turn out to be 74.42.

Now, I have the 2 sigma range of 74.42 to 95.98. Data within this range is considered typical, while anything outside could be an outlier.

 

Common Pitfalls and How To Avoid Them

Ensuring Accurate Data Entry Before Calculation

Before diving into calculations in Excel, ensuring the accuracy of data entry is a pivotal step that can’t be underscored enough. The old adage of “garbage in, garbage out” holds especially true when it comes to statistical analysis.

As a starting point, meticulously verify that each numeric entry is formatted correctly, as Excel distinguishes between numbers and text, and will exclude text from any numerical formulas. This means numbers should look like numbers, not dates, and certainly not text.

Additionally, take the time to comb through the data for any errors, such as typos, which can not only throw off your calculations but also fundamentally alter your analysis’s outcomes. Erroneous data can be particularly insidious as they may not always stand out at first glance.

Reviewing datasets for extreme outliers is also a critical practice. Such anomalies could either indicate an error in data collection or a rare event that should be separately categorized or considered in the analysis.

In cases where these checks reveal inconsistencies, we must clean our dataset. This may involve decision-making processes to correct, remove, or otherwise adjust outlier data points, and can often require revisiting the data source for validation.

Taking these cautionary steps before calculation not only aids in generating accurate results but also bolsters confidence in the reliability and integrity of our analysis.

Troubleshooting: When Results Don’t Make Sense

Facing perplexing outcomes in Excel is not uncommon in the realm of data analysis. Sometimes, after entering our well-rehearsed standard deviation formulas – be it =STDEV.S for a sample or =STDEV.P for a population – the results just don’t align with our expectations. It’s in these instances that our detective cap comes on, and we embark on a troubleshooting odyssey.

The first port of call is to double-check data formatting. Excel needs to decipher the range as numerical values, so ensure that no accidental text entries have slipped through the cracks. Beyond formatting, outliers warrant a thorough review. These extremes can unduly skew the standard deviation since it is a measure highly sensitive to variations in data.

One must also evaluate the correct application of functions – did we mistakenly use STDEV.P when STDEV.S was the appropriate choice for our sample data, or vice versa? Using the wrong function can set us off on a wild goose chase for misaligned outcomes.

When recalibration of formulas with these checkpoints still doesn’t lead to results that make sense, we may need to consider the possibility of an irregular distribution or a need for a more sophisticated statistical approach. Delving into the data with pivot tables or employing Excel’s Data Analysis Toolpak might reveal hidden patterns or call for a different strategy in analyzing variability.

It’s all about methodically verifying each step and being open to reassessing our dataset’s integrity. Only then can we systematically resolve the riddle of nonsensical results and reaffirm the robustness of our data analysis.

 

Real-World Applications of 2 Sigma

Quality Control and Consistency in Manufacturing

In the manufacturing sector, the application of 2 sigma is operatively intertwined with quality control and the drive for uncompromising consistency. Here’s where standard deviation really starts working its magic. By tracking the standard deviation, specifically the 2 sigma range, of the dimensions of manufactured components, businesses can assess whether their products are within the required tolerances.

Take an assembly line churning out engine parts. A consistently low standard deviation indicates that each component is being produced to near-identical specifications, with little variation. This precision is testimony to the effective and controlled operation of the manufacturing process. Conversely, a high standard deviation might signal that something’s amiss—tools could be worn down, or machinery might require recalibration.

Real-world benefits of applying 2 sigma in this context include reduced waste due to fewer defective products, increased customer satisfaction through quality assurance, and, ultimately, enhanced reputation and market competitiveness. Implementing this statistical monitoring ensures a systematic evaluation of production, pinpointing where improvements can be made before minor inconsistencies escalate into major issues. It is a proactive rather than reactive approach to quality management, integral in delivering excellence and reliability through every product that rolls off the line.

Risk Assessment in Financial Markets

In the maelstrom of financial markets, wielding the power of 2 sigma is akin to harnessing a navigational tool through turbulent waters. Risk assessment here revolves around the standard deviation to measure the volatility of investment returns, where a higher standard deviation indicates a greater potential risk.

For instance, when evaluating mutual funds, analysts pore over the 2 sigma range to discern the level of stability or tumult one can expect in returns. A mutual fund report brandishing a high 2 sigma value would suggest a higher propensity for value swings, signposting a riskier investment. In contrast, a lower 2 sigma implies a steadier, more predictable performance.

The application of standard deviation in this sector is dual-faceted – it assists in setting risk thresholds for portfolios and acts as a guide for diversification strategies to hedge against market volatility. Additionally, by back-testing historical data, financial analysts simulate various market scenarios – like the Global Financial Crisis of 2008 – to anticipate how current portfolios might respond to similar shocks. This type of forward-looking analysis steeped in historical context is instrumental in sculpting robust, resilient investment strategies that can withstand the caprices of the market. It is an indispensable ally in the financial analyst’s arsenal, underpinning decisions with the rigor of quantifiable risk measures.

 

FAQs

What is 2 sigma calculation?

A 2 sigma calculation refers to finding two standard deviations from the mean of a dataset. It represents a statistical measure that encompasses about 95% of data points under a normal distribution. This calculation serves to determine the variability and consistency of data, making it essential for quality control, risk management, and other analytical insights.

How do I calculate 2 standard deviations in Excel?

First, calculate the mean using =AVERAGE(), and the standard deviation with =STDEV.S(). Then, find 2 sigma by adding or subtracting two times the standard deviation from the mean using formulas like =Mean + 2*Sigma.

What role does standard deviation play in data analysis?

Standard deviation measures data variability around the mean. It helps determine how tightly data points are clustered and is essential for identifying consistency in datasets, such as financial risks or product quality.

What are some common mistakes when calculating 2 sigma in Excel?

Common pitfalls include incorrect data entry, using the wrong standard deviation formula (e.g., confusing STDEV.P with STDEV.S), and overlooking outliers, which can distort results. Double-check your data for accuracy.

What are real-world applications of 2 sigma?

In manufacturing, 2 sigma helps ensure product consistency, while in finance, it measures volatility to assess investment risks. It provides a statistical basis for quality control and risk management decisions.