The Ultimate Guide to Cumulative Relative Frequency in Excel

In my data analysis work, I’ve often found cumulative relative frequency helpful when tracking trends, distributions, and the accumulation of data over a range. It’s especially useful for understanding the proportion of each data point relative to the total, and how each new value builds upon the previous ones. In this article, I’ll walk you through calculating cumulative relative frequency in Excel step by step.

Key Takeaways:

• Cumulative relative frequency helps in tracking data trends by showing the accumulation of each data point relative to the whole.
• It is calculated by dividing each cumulative frequency by the total data points, often expressed as a percentage.
• Creating a cumulative frequency table in Excel is straightforward, starting with an organized dataset and using the SUM function.
• Applications of cumulative relative frequency range from educational assessment to financial and market analysis.
• Common pitfalls include confusing cumulative with relative frequency and using incorrect data ranges; double-checking formulas helps avoid these errors.

 

Introduction to the Art of Excel Analytics

Unveiling Cumulative Relative Frequency

When I first delved into the world of Excel analytics, I discovered the potent tool of cumulative relative frequency. It is a concept that reveals insights into data distribution, helping us see not just individual category frequencies, but their running total in relation to the whole set of observations.

Why Mastering This Skill Is Essential

Mastering cumulative relative frequency in Excel is crucial for anyone who needs to understand data patterns and trends. Whether you’re in academia, finance, marketing, or any field that involves data analysis, this skill allows for the identification of outliers, understanding of distributions, and informed decision-making.

By integrating cumulative relative frequency into your analytics toolkit, you can provide more nuanced interpretations of datasets, distinguishing you as a meticulous and skilled analyst.

The Essentials of Cumulative Relative Frequency

Understanding Key Concepts and Definitions

Before diving into calculations, it’s important to grasp the fundamental concepts. Cumulative relative frequency is not just an addition of numbers; it’s the sum of individual frequencies divided by the total number of data points, expressed as a running total.

This gives you a proportion that shows the accumulation of frequencies up to a certain point within your dataset. Understanding this helps in interpreting data more effectively, as you’ll be able to identify which portions of your data contribute to half, or perhaps even 75 percent, of your total observations.

The Role of Cumulative Relative Frequency in Data Analysis

In data analysis, cumulative relative frequency aids in understanding the spread and patterns within a dataset. It helps pinpoint where the bulk of data points lie in comparison to the whole. For example, educators can track cumulative test scores to identify performance trends, or businesses can analyze sales data to determine when they reach certain sales milestones.

By utilizing cumulative relative frequency, analysts can forecast probabilities, make informed predictions, and set performance benchmarks, ensuring comprehensive insights are derived from the raw data.

 

Step-by-Step Guide: Calculating Cumulative Relative Frequencies

Preparing Your Dataset for Analysis

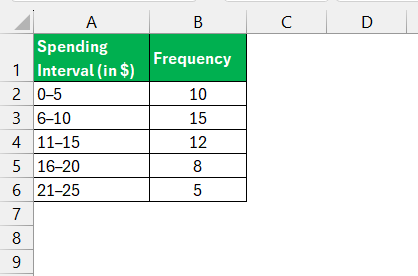
Preparing your dataset is an essential first step. We start by organizing the data into a clear, concise format. Ensure you have a column for the individual data categories and another for the frequency of occurrences in each category.

Cleanse your data by removing duplicates, correcting errors, and cross-verifying for accuracy.

Once your dataset is organized and clean, you can proceed to construct frequency tables, which are pivotal in running your cumulative relative frequency analyses in Excel.

Crafting a Cumulative Frequency Table with Ease

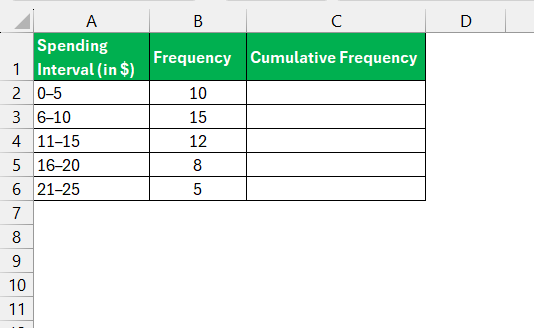
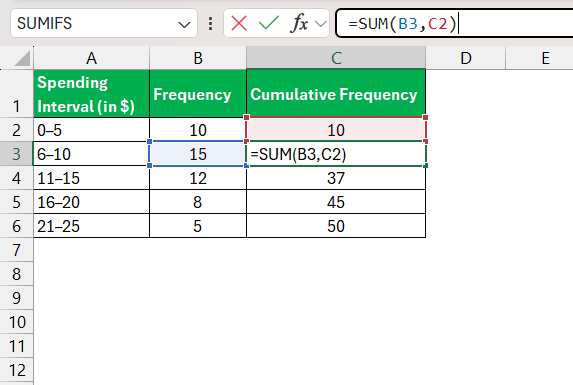
Once your data is in place, crafting a cumulative frequency table is quite straightforward. First, insert an additional column in your Excel spreadsheet next to the frequency column and title it “Cumulative Frequency”.

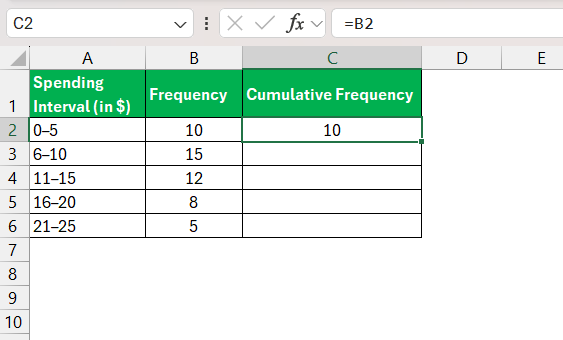
Start from the top of this new column; for the first row, the cumulative frequency equals the frequency.

For subsequent rows, it’s the sum of the previous cumulative frequency and the current frequency. To make things even simpler, you can use Excel’s SUM function—just be mindful to adjust the range appropriately as you go down the rows.

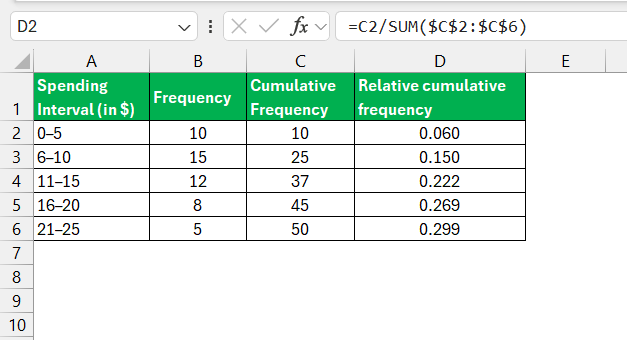
After tallying all the cumulative frequencies, you might choose to add a column for Relative cumulative frequency, where each cumulative frequency is divided by the total sum, converting it into a percentage if desired.

 

Practical Applications and Examples in Excel

Real-World Scenarios: From Classroom to Boardroom

The application of cumulative relative frequency extends into every domain where data is utilized to make strategic decisions. In educational settings, it can be used to track the progression of student grades over time. In the boardroom, these insights can direct financial decision-making by showing the cumulative percentage of revenue achieved per week or month.

Supply chain analysts can forecast product demands, while public health officials can track disease incidence rates. The versatility of this tool is what makes it indispensable in real-life situations, allowing professionals to translate raw data into actionable intelligence.

Common Missteps and How to Avoid Them

One common misstep in working with cumulative frequencies in Excel is mistaking it for simple frequency or relative frequency. This error can lead to inaccurate insights, as cumulative relative frequency looks at the aggregate effect over the data range. To avoid this, double-check your formulas and the range of cells included in the calculations.

Another pitfall is incorrect data range selection, which skews the cumulative total. Ensure that your cell references are absolute where needed, especially when copying formulas across multiple cells. Keeping vigilant about data entry and cleansing is also vital to avoid inaccuracies from the get-go.

 

Frequently Asked Questions

What is the cumulative relative frequency?

Cumulative relative frequency is the accumulation of previous frequencies up to a certain point, helping to understand how many observations fall below a particular value. It’s depicted as a running total and often expressed as a percentage, providing quick insights into the data distribution.

How do you find the cumulative relative frequency in Excel?

To find the cumulative relative frequency in Excel, first, calculate the relative frequency for each data item. Then, in a new column, add the relative frequencies of all previous items to the current one’s relative frequency. The last entry will equal one, signifying that 100% of the data has been accounted for.

How do you accurately calculate cumulative relative frequency in Excel?

To accurately calculate cumulative relative frequency in Excel, you need to ensure the initial data is correct. Then, use the COUNTIF and SUM functions to determine frequencies and relative frequencies. For the cumulative part, start with the first item’s relative frequency and add each subsequent item’s relative frequency to the cumulative total of the prior items. Use absolute cell referencing when necessary to maintain consistency across your calculations.

What are some common errors to watch out for when working with cumulative frequencies in Excel?

Common errors include incorrect range selection, which can lead to skewed results, and not using absolute references in formulas, which can cause errors when copying formulas down a column. To avoid these, carefully select the correct cell range and lock cell references, respectively using dollar signs, like $A$1, in your formulas.

What is the relative frequency formula in Excel?

The relative frequency formula in Excel is straightforward: You take the frequency of an occurrence divided by the total number of data points. If you have the frequency in cell B2 and the total number in cell B10, the formula in Excel would be =B2/B10. For accuracy, ensure cell B10 is an absolute reference when applying the formula to multiple cells.