Excel Bond Valuation Calculator – Step by Step Guide

Bond valuation is an essential tool for anyone involved in finance, whether you’re a personal investor, a financial analyst, or a portfolio manager. Understanding bond valuation helps us determine if a bond is fairly priced, overvalued, or undervalued, based on its expected cash flows and market conditions. I frequently use Excel for bond valuation because it’s flexible, easy to use, and offers numerous built-in financial functions that make calculating bond prices straightforward.

In this article, I’ll break down the bond valuation process step by step, explaining the key factors involved and showing you how to use Excel to determine a bond’s value. This guide will cover the theoretical aspects of bond valuation, explain the key inputs required, and provide examples of how you can implement bond valuation in Excel.

Key Takeaways:

• Bond valuation helps determine the fair price of a bond by calculating the present value of future cash flows, including coupon payments and face value.
• Key components needed for bond valuation include face value, coupon rate, payment frequency, time to maturity, and the market interest rate (discount rate).
• Present value is the core concept in bond valuation, where future payments are discounted to reflect their worth today.
• Excel offers built-in functions, such as PV, to calculate the present value of coupon payments and the bond’s face value.
• A bond’s value is the sum of the present value of both its coupon payments and face value, helping investors assess if a bond is underpriced or overpriced.

 

Introduction to Bond Valuation Calculator

What is Bond Valuation?

At its core, bond valuation is the process of determining the present value of a bond’s future cash flows. These cash flows consist of periodic coupon payments (if it’s a coupon bond) and the repayment of the bond’s face value (principal) at maturity. The key idea is to discount these future payments back to the present using the current market interest rate, or discount rate, to arrive at the bond’s fair market price.

In other words, bond valuation helps us understand what a bond is worth today, based on what it promises to pay in the future. This is critical in making investment decisions, as it tells us whether we are paying too much or too little for a bond.

Key Components of Bond Valuation

To perform bond valuation, we need several important pieces of information:

• Face Value (or Par Value): This is the amount the bondholder will receive at maturity. It’s usually $1,000 for corporate bonds.
• Coupon Rate: The interest rate the bond pays, expressed as a percentage of the face value. For instance, a 5% coupon rate on a $1,000 bond means the bondholder will receive $50 in interest annually.
• Coupon Payment Frequency: Bonds usually pay interest either annually or semi-annually. Understanding the payment frequency is important when calculating the bond’s value.
• Time to Maturity: The number of years (or months) until the bond matures and the face value is repaid.
• Market Interest Rate (Discount Rate): This is the rate of return required by investors in the current market, given the bond’s risk level. It is the key rate used to discount the bond’s future cash flows.

Understanding Present Value and Discounting

The concept of present value is fundamental to bond valuation. The idea is that money you receive in the future is worth less than the same amount received today because money today can be invested and earn interest. The process of calculating the present value involves discounting the future cash flows back to the present using the market interest rate.

In the case of bonds, we discount both the coupon payments and the face value to the present, summing them to get the bond’s total value.

 

Using Excel for Bond Valuation

Now, let’s get into the details of how to use Excel for bond valuation. Excel has several built-in functions that make bond valuation easy, and I’ll show you how to use them step-by-step.

Setting Up the Inputs in Excel

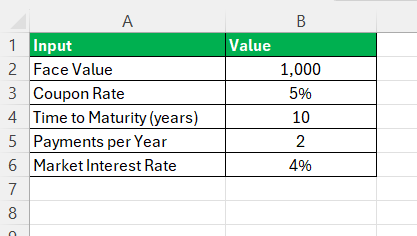
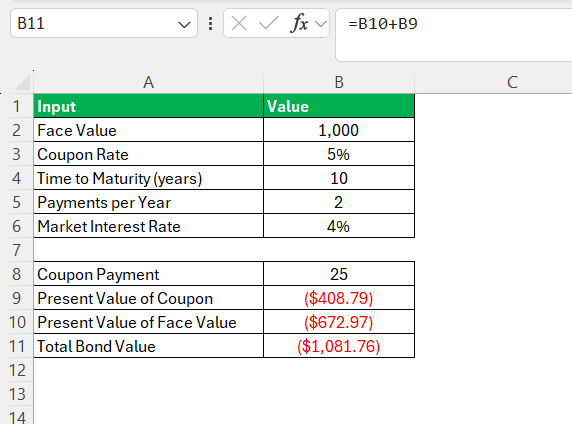
Before performing any calculations, it’s essential to set up the key inputs in an Excel spreadsheet. Let’s assume we have a bond with the following characteristics:

• Face Value: $1,000
• Coupon Rate: 5%
• Time to Maturity: 10 years
• Payment Frequency: Semi-annual (twice a year)
• Market Interest Rate: 4%

Calculating the Coupon Payments

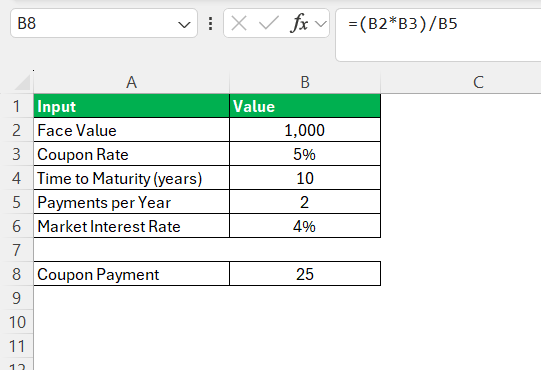
The first step is to calculate the amount of each coupon payment. The coupon payment is simply the face value multiplied by the coupon rate, divided by the number of payments per year.

In Excel, you can use the following formula to calculate the semi-annual coupon payment:

= (Face Value * Coupon Rate) / Payments per Year

In our example, this would be:

So, the bondholder will receive $25 every six months.

Calculating the Present Value of Coupon Payments

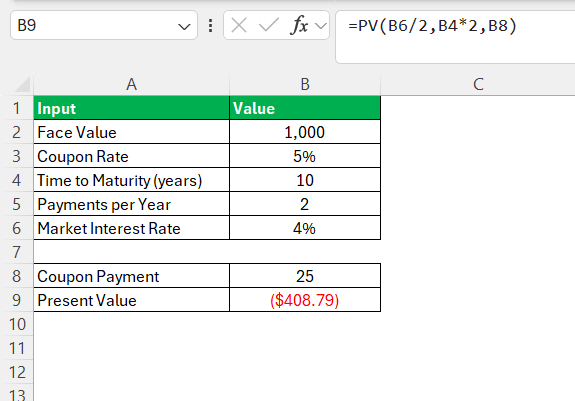
The next step is to calculate the present value of the coupon payments. We’ll use Excel’s PV (Present Value) function to do this. The syntax of the PV function is:

=PV(rate, nper, pmt, [fv], [type])

Where:

• rate: The market interest rate per period. In this case, it’s the annual market interest rate divided by the number of payments per year.
• nper: The total number of periods (years to maturity multiplied by payments per year).
• pmt: The coupon payment per period.

For our example, the formula to calculate the present value of the coupon payments would look like this:

This gives the present value of the bond’s coupon payments.

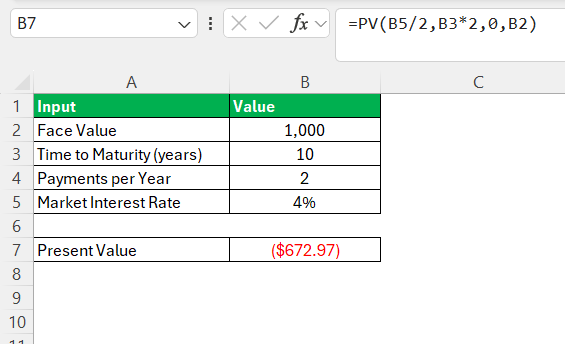
Calculating the Present Value of the Face Value

The bondholder will also receive the face value ($1,000) at maturity, so we need to calculate the present value of the face value using the same PV function. The formula in Excel would be:

This calculates the present value of the $1,000 that the bondholder will receive in 10 years.

Summing the Results

The bond’s value is the sum of the present value of the coupon payments and the present value of the face value. You can sum the two PV calculations in Excel to get the total bond price.

For our example, the total bond value is:

This gives the bond’s theoretical fair value in today’s terms.

 

Additional Considerations

Premium vs. Discount Bonds

When the market interest rate is lower than the bond’s coupon rate, the bond will trade at a premium (above par value). Conversely, if the market interest rate is higher than the coupon rate, the bond will trade at a discount (below par value). Excel allows us to model both scenarios easily by adjusting the market interest rate input.

Zero-Coupon Bonds

For zero-coupon bonds, there are no periodic coupon payments. The bondholder only receives the face value at maturity. In this case, the bond’s value is simply the present value of the face value.

You can calculate this in Excel by setting the coupon payment to zero in the PV function.

 

FAQ on Excel Bond Valuation

What is the formula for bond valuation?

The formula for bond valuation is the Present Value of Future Payments divided by the appropriate discount rate, which is termed as Yield to Maturity (YTM). Specifically:

Bond Value = PV of Interest Payments + PV of Face Value

How to calculate the value of a bond using Excel?

To calculate the value of a bond using Excel, use the PV function to find the present value of the coupon payments and the face value. The formula structure is:

=PV(rate/nper, nper, payment, fv)

Where ‘rate’ is the discount rate/YTM, ‘nper’ is the number of periods, ‘payment’ is the coupon payment, and ‘fv’ is the face value.

Alternatively, the PRICE function can be used for a more complete bond valuation, taking into consideration the timing of the coupon payments.

How Do I Use the PRICE Function Specifically for Bonds?

To use the PRICE function specifically for bonds in Excel:

=PRICE(settlement, maturity, rate, yld, redemption, frequency, [basis])

• ‘settlement’ is the date the buyer acquires the bond.
• ‘maturity’ is when the bond expires.
• ‘rate’ is the bond’s annual coupon rate.
• ‘yld’ is the bond’s annual yield.
• ‘redemption’ is the value at maturity per $100 of face value.
• ‘frequency’ indicates the number of coupon payments per year.
• ‘basis’ (optional) defines the day count convention to use.

Ensure the dates are in Excel’s date format and rates are represented as decimals.

What Are the Differences Between PV, FV, and PRICE When Valuing Bonds?

The PV (Present Value) function calculates the current value of a series of future cash flows, including bond coupon payments and principal repayment, at a certain discount rate. The FV (Future Value) function, on the other hand, projects the value of an investment at a future date based on periodic, constant payments and a constant interest rate.

The PRICE function differs because it calculates the bond’s price per $100 of face value, considering the expected yield, and is specifically designed for bonds. It accounts for the timing of coupon payments and the term until maturity, providing a clean bond price.

These functions cater to different aspects of bond valuation based on the financial data and outcomes desired.

Can Excel Calculate Bond Valuation on Any Given Date?

Yes, Excel can calculate bond valuation on any given date using functions such as PRICE or PV, as long as you provide the correct settlement date and other required parameters.