The Ultimate Guide to Standard Deviation as a Percentage in Excel

Understanding standard deviation in Microsoft Excel empowers you to quickly assess the variability of your data around the mean. This statistical measure, which may seem complex at first, becomes manageable with Excel’s powerful functions. Knowing how to calculate and interpret standard deviation as a percentage can greatly enhance your data analysis capabilities, allowing for more informed decision-making.

Key Takeaways

1. Definition and Importance: Standard deviation measures the dispersion of data points around the mean, essential for understanding data variability.
2. Excel Functions: STDEV.S and STDEV.P are key Excel functions for calculating standard deviation, suitable for sample and population data, respectively.
3. Practical Steps: Calculating standard deviation in Excel involves entering data, selecting the appropriate function, and interpreting the results.
4. Percentage Standard Deviation: Converting standard deviation to a percentage provides a relative measure of variability, useful for comparing datasets with different means.
5. Real-Life Applications: Standard deviation is vital in fields like stock market analysis, quality control, and education to assess risk, consistency, and performance.

 

Introduction

Demystifying Standard Deviation in Excel

Imagine being able to swiftly identify how much your data fluctuates around the mean—essentially gauging the pulse of your dataset. That’s the power of understanding standard deviation! What might sound like a complex mathematical concept becomes far less intimidating when you harness Excel to do the heavy lifting for you.

Why Understanding Percentage Standard Deviation Matters

Grasping the concept of percentage standard deviation equips you with a versatile tool for data analysis. It transcends mere numbers and presents a relative measure of variability, which is incredibly useful when comparing datasets with differing averages. Think of it as your data’s heartbeat in percentage form, giving you insights into the rhythm and consistency of the information you’re examining.

 

The Basics of Standard Deviation

What is Standard Deviation and Why Use It?

Standard deviation is the mathematical compass pointing to the spread of your data. When you calculate it, you’re measuring the dispersion of dataset figures around their mean (average). If you’re navigating through a sea of numbers, the standard deviation is your North Star – guiding you to understand the volatility and consistency of data.

It’s a must-have for anyone looking to make predictions, establish quality controls, or manage financial portfolios because it translates abstract numbers into actionable insights.

The Difference Between Sample and Population Standard Deviation

When you’re analyzing data, it’s crucial to know whether you’re looking at a sample of the data or the entire population. The population standard deviation includes every single data point in the set, providing an exact measure of spread. It’s the go-to when you have full access to the data you’re interested in.

On the other hand, sample standard deviation gives you an estimate based on a portion of the population. It compensates for the smaller group size by tweaking the formula slightly, making the standard deviation a bit larger to more accurately reflect the potential variability in the entire population. Each standard deviation has its own scenario, so choosing the right one helps ensure your analysis is on point!

 

Calculating Standard Deviation in Excel

Key Functions: STDEV.S vs. STDEV.P

Let’s zoom in on the crux of the matter: STDEV.S and STDEV.P are Excel’s duo of functions tailored for calculating standard deviation. They each have specializations: STDEV.S is your go-to when you have a snippet—a sample of a larger populace, while STDEV.P steps in when the whole population’s data is at your fingertips.

Remember, both functions help you quantify how spread out your data is, but it’s crucial to pick the one that matches your dataset. If you select STDEV.S, your standard deviation will consider that there’s more data out there, adjusting itself to reflect that uncertainty. STDEV.P, however, assumes you’ve seen the full picture, giving a more precise look at the variability.

Step-by-Step: Calculating Standard Deviation in Numbers

Calculating standard deviation in Excel is like following a recipe, and you don’t need to be a math chef to whip it up! Here’s a quick culinary guide:

STEP 1: Enter Your Data: Like picking your ingredients, get your data neatly arrayed in a column or row.

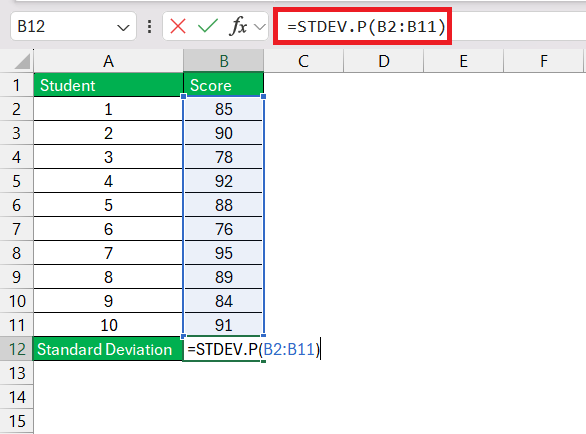
STEP 2: Enter the Formula: Click the cell where you desire the result, type ‘=’ followed by your selected function, and enclose the range of your data within parentheses. For example, “=STDEV.P(B2:B11)”.

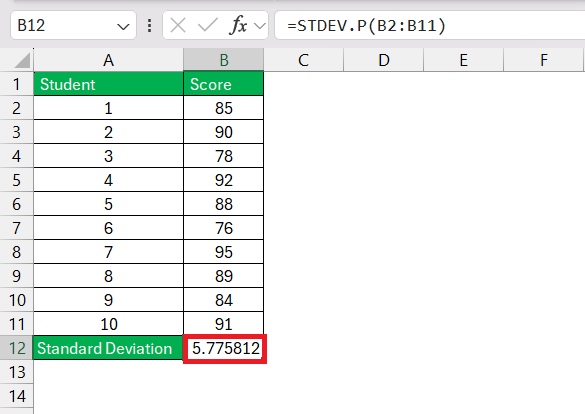
STEP 3: Execute and Serve: Hit enter and voila, Excel calculates the standard deviation for your dataset.

Remember, following these steps correctly ensures you get a meaningful measure of how varied your data is. An incorrectly calculated standard deviation could result in you misinterpreting the flavors—uh, I mean data patterns.

 

Converting Standard Deviation to a Percentage

Understanding the Significance of Percentage in Data Analysis

When you’re sifting through data, a percentage can be your lighthouse, guiding you through a fog of numbers. It standardizes the variability, giving it context and making it easy to compare across different sets. It’s all about relativity; when you calculate the standard deviation as a percentage, you tap into understanding not just the raw spread, but also how significant that spread is relative to the average.

In essence, it’s the difference between knowing there’s a storm and understanding how it really affects your voyage on the sea of data.

How to Calculate Standard Deviation as a Percentage in Excel

Calculating the percentage standard deviation in Excel might sound daunting, but it’s truly a breeze with the right formula. Essentially, you’re taking the standard deviation and placing it on a balanced scale with the mean to gauge its relative weight as a percentage. Here’s the treasure map:

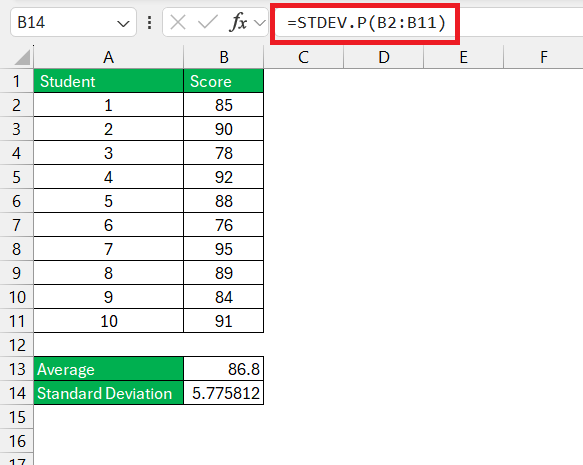
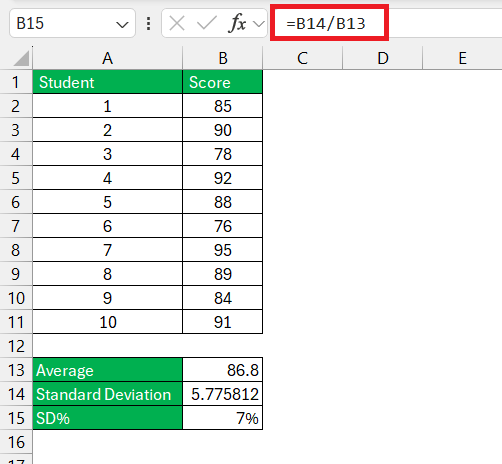
• Calculate Average: Enter the average formula =AVERAGE(B2:B11)

• Calculate Standard Deviation: Enter the standard deviation formula =STDEV.P(B2:B11)

• Craft the Percentage Formula: In a new cell, type in “=(Standard Deviation cell reference/AVERAGE(data range))”. This magical incantation transforms your standard deviation into a percentage.

Executing this formula hands you a powerful lens to scrutinize your data’s variability in a way that resonates clear as a bell, no matter the dataset size.

 

Real-Life Applications

Using Percentage Standard Deviation for Risk Assessment

Risk assessment loves percentage standard deviation; they’re like a dynamic duo of data analysis. By converting standard deviation to a percentage, you get to put on X-ray goggles, seeing through the raw data to focus on how variation might impact your project or investment.

A higher percentage points to higher risk—it means data points are playing fast and loose around the average. Alternatively, a lower percentage suggests a steadier, more predictable set of outcomes, ideal for when consistency is key.

Enhancing Quality Control with Percentages

In the realm of quality control, percentages are the ace in your pocket. By using percentage standard deviation, you can compare the uniformity of products or processes, regardless of their scale or unit of measure. It’s like having a universal language for consistency across your operation. Spotting a low percentage? You’ve got a process that’s tighter than a drum. If that percentage starts climbing, however, it’s a red flag that something’s off-kilter and needs attention pronto.

Businesses tracking component dimensions, for example, find solace in low and stable percentage standard deviations, signaling a process that’s under control and products that hit the mark every time.

 

Tips and Tricks

Avoiding Common Mistakes When Working with Standard Deviation

Strolling through the garden of standard deviation calculations, it’s easy to trip over a few hidden roots. A classic mistake? Mixing up STDEV.S and STDEV.P – it’s like confusing the salt for sugar, and your data dessert will taste a bit off. Also, watch out for outliers that can skew your interpretation—imagine a stray cat crashing a dog show. Lastly, neglecting to clean your data before diving in can lead to a bramble patch of issues later on. Keep these common pitfalls in mind, and your path to accurate data analysis will be as clear as a well-maintained trail.

Dynamic Calculations with Tables & Named Ranges

Want to make your standard deviation calculations as adaptable as a chameleon? Turn to Excel Tables and Named Ranges! These features transform your static formulas into dynamic acrobats that automatically adjust as your data grows or changes.

With Excel Tables, adding new data is a breeze, and the standard deviation formula you’ve fashioned will auto-expand to incorporate the fresh figures. Named Ranges are equally nifty; define the scope of data once, and any changes in that range are immediately reflected in your standard deviation calculation. It’s like having a smart assistant who keeps your analysis up to speed, making sure no stone—or data point—is unturned.

 

Visual Representation of Data

Creating Charts for a Better Understanding of Data Variability

Excel Charts are like the storybooks of the data world, turning rows and columns of numbers into visual tales. When you’re trying to tease out the nuances of data variability, a well-crafted chart can be worth a thousand cells.

For example, a line chart can track the ebbs and flows of your data over time, while a scatter plot can reveal the relationship—or lack thereof—between two variables. By portraying standard deviation on these charts, you give your audience a clear, immediate sense of how spread out the data is. The result? A compelling narrative that makes the invisible patterns of your data dance before your eyes.

Adding Standard Deviation Error Bars

Whether you’re presenting to a room of seasoned analysts or crafting a report for stakeholders, clarity is key. Standard deviation error bars are the heavy artillery in your Excel arsenal, visually hammering home the variability of your data directly on the chart.

Here’s the artillery setup:

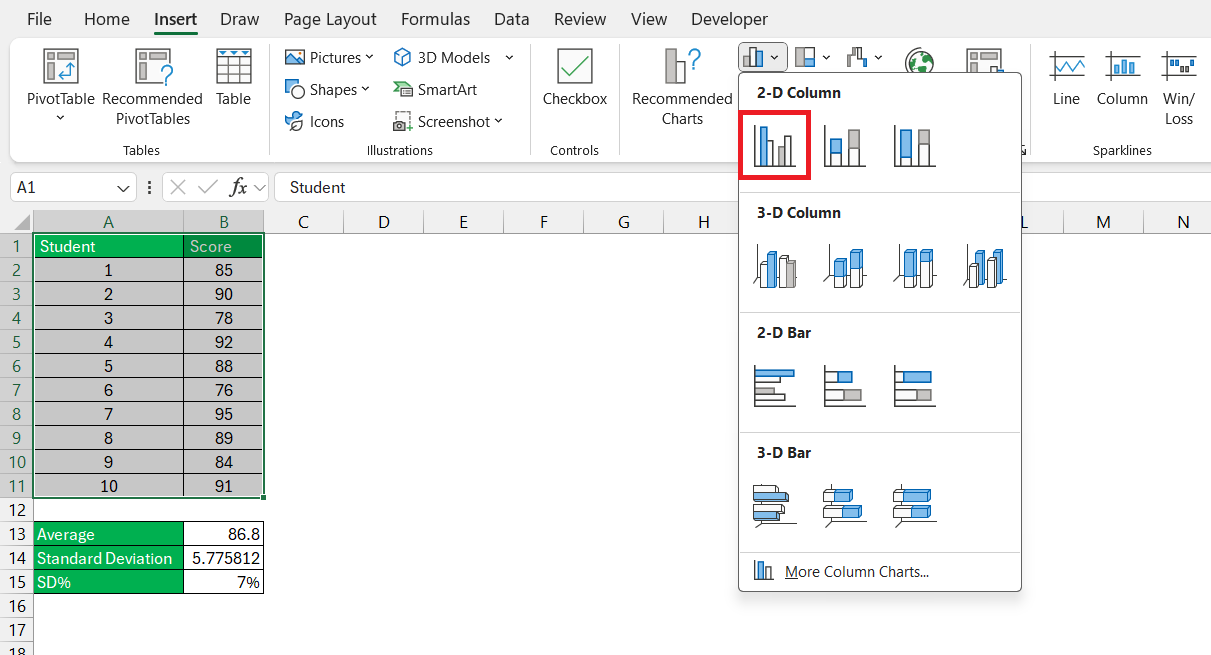
STEP 1: First, create your chart by selecting your data and choosing the best chart type from the ‘Insert’ tab.

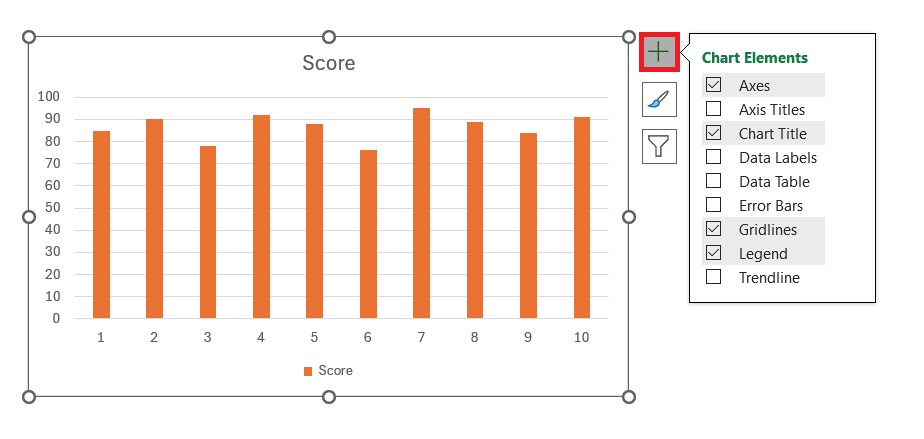
STEP 2: Click on the freshly minted chart and look for ‘Chart Elements’.

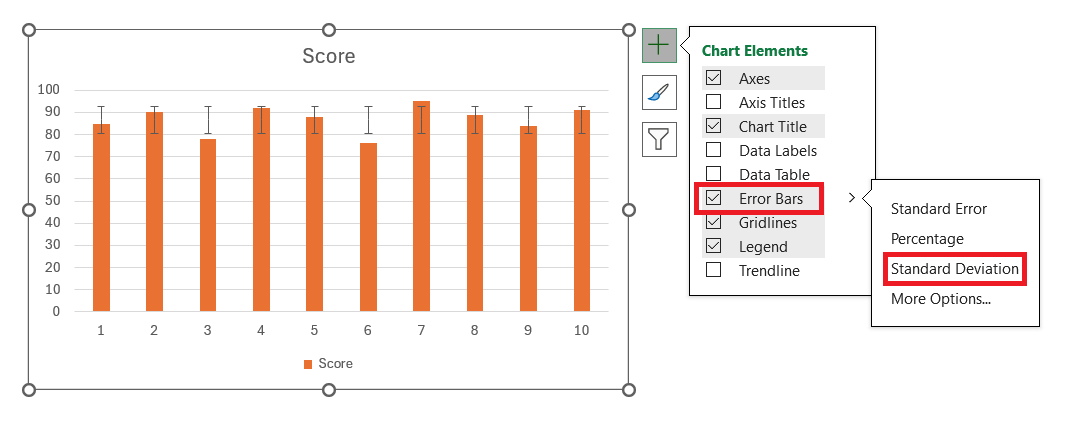
STEP 3: Dive into ‘Error Bars‘, and select ‘Standard Deviation’ to blanket your data points with bars that represent variability.

With error bars in place, you’re not just telling your audience about the data’s stability or volatility; you’re showing them, making your data’s story that much more persuasive.

 

FAQs

How to do percent standard deviation in Excel?

To calculate the percent standard deviation in Excel:

• Compute the standard deviation with “=STDEV.S(range)” for a sample or “=STDEV.P(range)” for a population.
• Get the mean using “=AVERAGE(range)”.
• Then use “=(STDEV/MEAN)*100” to find the percentage.

This provides the relative standard deviation, a handy percentage showing how much your data deviates from the average.

How Do I Choose Between STDEV.S and STDEV.P When Calculating Standard Deviation?

Select the STDEV.S function when you’re working with a sample of a larger population; it factors in the sample’s limitations. Use STDEV.P if you’ve got data for the entire population, as it assumes no data is missing. Choosing the right one ensures your findings accurately reflect your data’s dispersion.

What Is the Formula for Percentage Standard Deviation in Excel?

The formula for percentage standard deviation, also known as Relative Standard Deviation (RSD), in Excel is: “= (STDEV(range)/AVERAGE(range))”. Replace ‘range’ with your data set’s cell range to get the standard deviation as a percentage of the mean.

How to convert standard deviation into percentage?

To convert the standard deviation into a percentage, you divide the standard deviation by the mean (average) of the data set. This percentage gives you the standard deviation relative to the mean, providing a dimensionless measure of variability.

Which SD is used?

The choice between “sd” depends on the nature of your dataset:

• STDEV.S (Sample Standard Deviation): Use this when you’ve sampled part of a population.
• STDEV.P (Population Standard Deviation): Use this when you have data for the whole population.

Picking the correct “sd” impacts the accuracy of your variability measurement.