Calculating the t-statistic in Excel is a crucial skill for anyone working with statistical data. Excel’s built-in Data Analysis Toolpak provides a straightforward way to perform t-tests, enabling users to compare sample means and assess hypotheses with ease. This article will guide us through the steps to calculate the test statistic using Excel.
Key Takeaways:
- Understanding the T-Test in Excel: Learn the purpose of a t-test and how it helps in comparing data sets.
- Enabling the Data Analysis ToolPak: Discover how to activate the ToolPak to perform t-tests in Excel.
- Running a T-Test: Follow step-by-step instructions to calculate the t-statistic using Excel’s Data Analysis tool.
- Interpreting Results: Understand how to interpret the t-statistic, p-value, and test significance.
- Troubleshooting and Limitations: Learn how to overcome common issues and limitations of Excel’s t-test functionality.
Table of Contents
Introduction to T-Test in Excel
What is a T-Test?
When I dive into data analysis, I might need to compare two sets of data to see if they’re statistically different. This is where a T-Test becomes useful. It’s a type of hypothesis test that helps me determine whether the means of two datasets come from different populations or if any observed difference is simply due to random chance.
Importance of Excel for Statistical Analysis
Excel is great for crunching numbers and organizing data, but it’s also a powerful tool for statistical analysis. With its built-in functions and analysis add-ons, Excel simplifies complex statistical processes, making them accessible even if I’m not a stats expert.
I can perform a wide range of tests, including T-Tests, and Excel’s intuitive interface and visual aids help me quickly interpret and share my findings. Essentially, Excel brings the power of statistical software right to my spreadsheets, making significant data analysis convenient and accessible.
Getting Started with Excel’s T-Test Tool
Preparing Your Data for T-Test
Before jumping into performing a T-Test in Excel, getting your data in tip-top shape is crucial. This means you’ll need to organize your data into a clean, orderly format.
It’s vital to remove any outliers or incorrect data points that could skew your results; these are often easier to identify once your data is neatly lined up. Also, make sure your data does not have any missing values, as this could invalidate your analysis.
Installing the Data Analysis ToolPak
Taking your Excel experience to the next level with statistical analysis is as simple as enabling the Data Analysis ToolPak. This free add-on is your gateway to powerful tools like the T-Test, expanding Excel’s capabilities beyond the basic functions.
STEP 1: To get started, open Excel and click on ‘File’, followed by ‘Options’.
STEP 2: Select ‘Add-ins’ and choose ‘Excel Add-ins’ from the drop-down menu. Click ‘Go’.
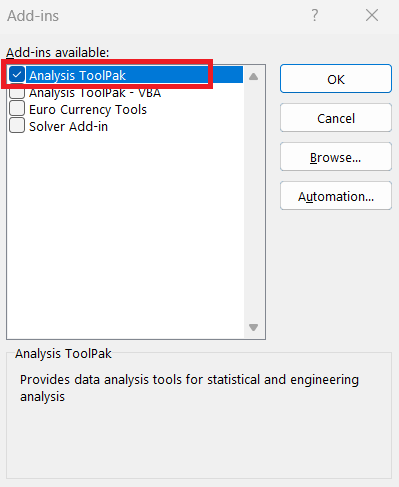
STEP 3: In the pop-up window, tick the checkbox for ‘Analysis ToolPak’ and click ‘OK’.
Voilà, you’ve just equipped Excel with a full kit of statistical instruments ready to analyze your data.
Running a T-Test in Excel
To carry out a one-sample T-Test, which essentially looks at whether the mean of a single sample diverges from a known value, follow these straightforward steps:
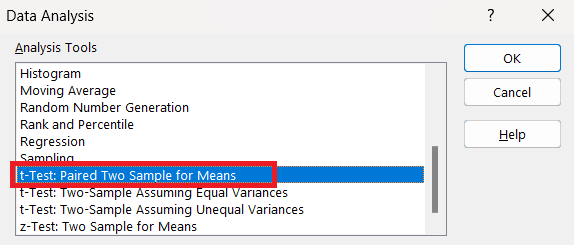
STEP 1: Click the ‘Data’ tab in Excel to reveal its ribbon. Access the ‘Data Analysis’ tool that you’ve previously activated from the ToolPak.
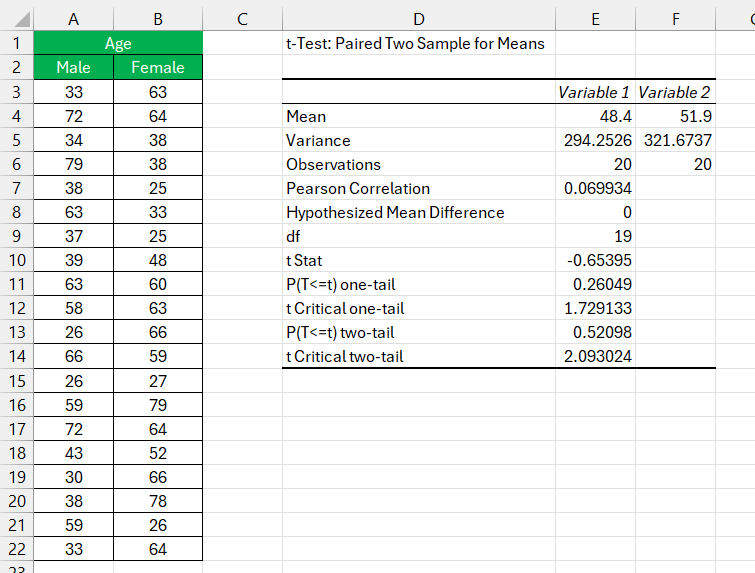
STEP 2: From the analysis options, select ‘t-Test: Paired Two Sample for Means’ and hit ‘OK’.
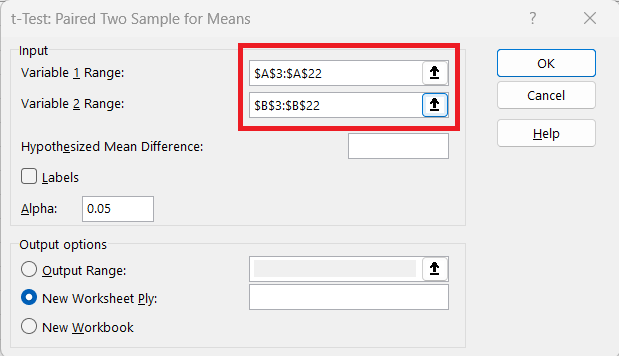
STEP 3: You will then fill out the dialog box ‘Input Range’ with the data range of your sample.
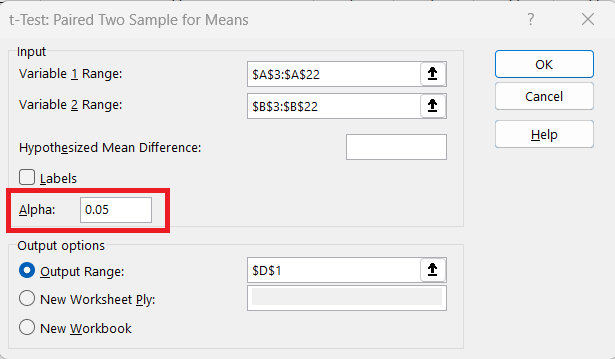
STEP 4: Input your ‘Alpha’ value, typically 0.05 for a 95% confidence level.
Excel does the heavy lifting, presenting you with the T-Test’s results, which reflect how much your sample mean differs from the population mean, assuming your data is properly prepped and error-free.
Interpreting T-Test Results in Excel
Understanding the Test Statistic Value
The test statistic value is essentially your calculated T-Statistic, and it’s a crucial number that helps you determine the significance of your test results. This value represents the ratio of the difference between the group means to the variation that exists within the groups. In simpler terms, it’s a measure of how far apart the groups are when taking into account the spread of scores within the groups.
When you’re looking at your test statistic value in Excel, a higher absolute value could indicate a more significant difference between the group means. However, you can’t make any conclusions based on the test statistic alone; you’ll need to compare it against a critical value from the T distribution to assess significance. This is where knowing your degrees of freedom — calculated from your sample size — and your chosen level of significance comes into play.
Making Sense of P-Value and Hypothesis Testing
The p-value tells a story about your hypothesis test in Excel – it quantifies how likely your test results are if the null hypothesis were true. In other words, it helps you understand the probability of stumbling upon the observed results by chance.
To decipher the p-value:
- Compare the p-value to your alpha level, usually set at 0.05. If it’s smaller, this suggests there’s enough evidence to reject the null hypothesis.
- A p-value larger than the alpha level means there isn’t enough evidence to do so.
Remember, a lower p-value isn’t just “better”—it means there’s a stronger case against the null hypothesis, giving credibility to your alternative hypothesis.
Making sense of hypothesis testing requires you to see the p-value not as a black-and-white verdict but as an indicator of evidence strength. The closer the p-value is to zero, the stronger the evidence against the null hypothesis. This nuanced understanding helps prevent overstating the results of your test while providing a reliable foundation for decision-making.
Troubleshooting Common Issues with Excel T-Tests
Overcoming Limitations of Excel’s T-Test Functionality
While Excel is a mighty tool for statistical analysis, it’s important to acknowledge its limitations with T-Tests. You might encounter confines such as the inability to handle non-numeric data or perform certain advanced tests. Plus, Excel’s algorithms are optimized for general use, so very specific or non-standard calculations might not be as precise as those done with specialized statistical software.
To overcome these limitations, consider the following tips:
- Familiarize yourself with the kinds of tests Excel can perform and understand the math behind them. This way, you’ll know the appropriateness of using Excel for your specific test.
- If you’re dealing with non-numeric data, preprocess it outside Excel before conducting a T-Test.
- Keep in mind that Excel’s T-Test assumes that your data is randomly sampled and follows a normal distribution; if this isn’t the case, the test results might not be valid.
- Looking for accuracy beyond what Excel can offer? Supplement Excel with more robust statistical packages for complex analyses.
Sometimes, stepping beyond Excel’s built-in functions into the realm of VBA scripting or integrating with other statistical tools will give you that cutting edge.
Frequently Asked Questions
How do you find the test statistic in Excel?
You can find the test statistic in Excel by using the T.TEST function, which calculates the t-statistic and p-value directly from your data. You can also use the T.INV or T.INV.2T functions for critical values.
Can Excel Perform All Types of T-Tests?
Yes, Excel can perform one-sample, two-sample (independent), and paired-sample t-tests using the T.TEST function or the Data Analysis Toolpak.
How Can I Ensure My Data is Suitable for a T-Test?
Ensure your data is normally distributed, the samples are independent, and the variances are equal or similar, especially for two-sample t-tests.
What to Do If the T-Test Results Are Inconclusive?
If your t-test results are inconclusive, consider increasing your sample size, checking for data errors, or using a non-parametric test like the Mann-Whitney U test.
When can I use one-tailed tests?
Use a one-tailed test when you have a specific directional hypothesis, predicting that one mean will be greater or less than the other.
John Michaloudis is a former accountant and finance analyst at General Electric, a Microsoft MVP since 2020, an Amazon #1 bestselling author of 4 Microsoft Excel books and teacher of Microsoft Excel & Office over at his flagship MyExcelOnline Academy Online Course.